Euler line (distance between the circumcenter and the orthocenter of a triangle)
Description
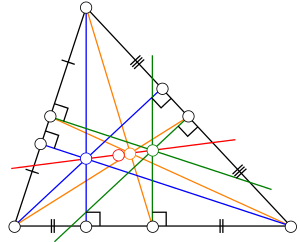
In geometry, the Euler line is a line determined from any triangle that is not equilateral. It passes through several important points determined from the triangle, including the orthocenter, the circumcenter, the centroid, the Exeter point and the center of the nine-point circle of the triangle. In any triangle, the orthocenter, circumcenter and centroid are collinear. The distance between the circumcenter and the orthocenter of a triangle is related to the circumradius and the sides of the triangle.
Variables
| OH | The distance between the circumcenter and the orthocenter of the triangle (m) |
| R | The circumradius of the triangle (radius of the circle which passes through all the vertices of the triangle) (m) |
| a | Side of the triangle opposite to angle A (m) |
| b | Side of the triangle opposite to angle B (m) |
| c | Side of the triangle opposite to angle C (m) |