Area of an arbitrary triangle (incircle and excircles)
Description
The incircle or inscribed circle of a triangle is the largest circle contained in the triangle; it touches (is tangent to) the three sides. The center of the incircle is called the triangle’s incenter and can be found as the intersection of the three internal angle bisectors. Excircle or exscribed circle of a triangle is a circle lying outside the triangle, tangent to one of its sides and tangent to the extensions of the other two. Every triangle has three distinct excircles, each tangent to one of the triangle’s sides. The triangle’s area is related to the inscribed radius and the excircles radii.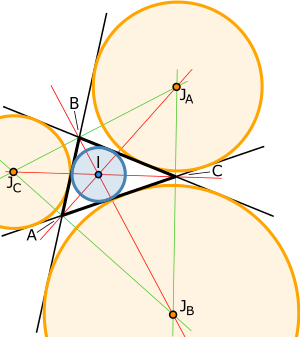
Variables
| A | Area of the triangle (m2) |
| r | Inscribed circle radius (m) |
| ra | Excircle radius (tangent to side a) (m) |
| rb | Excircle radius (tangent to side b) (m) |
| rc | Excircle radius (tangent to side c) (m) |